Question

In the figure below, BD and EC are diameters of circle P.

What is the arc measure of AE in degrees?

Answer 1

27°

Explanation:

We know by given

`m \angle APB = 90\°`

`m \angle DPE=63\°`

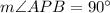
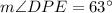
According to the given circle,

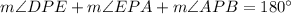`m\angle DPE + m\angle EPA + m\angle APB=180\°`, by supplementary angles.

Replacing each value, we have

`63\° + m\angle EPA + 90\° = 180\°\\m \angle EPA = 180\° - 153\°\\m \angle EPA = 27\°`

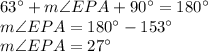
Now, the angle EPA subtends the arc AE, and this angle is a central angle. So, according to its defintion, the arc AE is equal to its central angle.

`arc(AE)= m\angle EPA = 27\°`

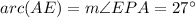
Therefore, the answer is 27°