Answer:
1. 11x + C
4. 5/14 x^2 + C
Explanation:
When taking an integral of a one term power function, which means it has one number multiplied by an x t some power, so ax^b you use the power rule for integration.
ax^b becomes
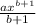
So first you identify a and b and just plug in. I will walk you through all of them through the comments if you like, but here I am just going to solve one. Inf act I'll solve one that looks the hardest.
Number 4. We have (5/7)x. so a is 5/7 and b is 1. so just fill in.

From here you can actually divide 5/7 by 2 and get 5/14 so the answer is 5/14 x^2 + c. Since these are indefinite integrals NEVER forget the constant C. Go ahead and try taking tis derivative though. Just remember C is a constant.
Also I wanted to mention, if you just have a number, so in number 1 it's ust 11, it is the same as 11x^0 so b is 0 in this case. So bonus, the answer to 11 is 11x + C. Never forget the C.
Let me know if there are any specifically you need help with, or you do want to work through all of them.