Question

F(x)=−2x+16
g(x)=4x+10
please give me the intersection points

Answer 1

(1, 14)

Explanation:

Given functions:

`\begin{cases}f(x)=-2x+16\\g(x)=4x+10\end{cases}`

Substitute the function notation for y to create a system of equations:

`\begin{cases}y=-2x+16\\y=4x+10\end{cases}`

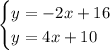
To find the points of intersection of the given functions, solve the system of equations.

Multiply the first equation (function f(x)) by 2:

`\implies 2y=-4x+32`

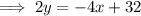
Add this to the second equation (function g(x)) to eliminate x:

`\begin{array}{r l}4x+10 & = y\\+ \quad -4x+32 & = 2y\\\cline{1-2} 42 & = 3y\end{array}`

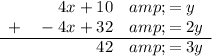
Solve for y:

`\implies 3y=42`

`\implies y=14`

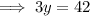
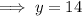
Substitute the found value of y into one of the equations and solve for x:

`\implies 4x+10=14`

`\implies 4x=4`

`\implies x=1`

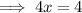
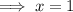
Therefore, the point of intersection of the given functions is (1, 14).

Answer 2

• (1, 14)

Explanation:

The intersection is when both functions have same coordinates

• f(x) = g(x)

Substitute to get

• - 2x + 16 = 4x + 10
• 4x + 2x = 16 - 10
• 6x = 6
• x = 1

The y- coordinate is

• y = - 2(1) + 16 = -2 + 16 = 14

So the intersection point is (1, 14)