Question

Let p equal the proportion of drivers who use a seat belt in a country that does not have a mandatory seat belt law. It was claimed that p = 0.14. An advertising campaign was conducted to increase this proportion. Two months after the campaign, y = 104 out of a random sample of n = 590 drivers were wearing their seat belts . Was the campaign successful? (a) Define the null and alternative hypotheses. (b) Define a rejection region with an α = 0.01 significance level. (c) Determine the approximate p-value and state your conclusion.

Answer 1

See explanation

Explanation:

Solution:-

- Let the proportion of drivers who use a seat belt in a country that does not have a mandatory seat belt law = p.

- A claim was made that p = 0.14. We will state our hypothesis:

Null hypothesis: p = 0.14

- Two months after the campaign, y = 104 out of a random sample of n = 590 drivers were wearing their seat belts. The sample proportion can be determined as:

Sample proportion ( p^ ) = y / n = 104 / 590 = 0.176

- The alternate hypothesis will be defined by a population proportion that supports an increase. So we state the hypothesis:

Alternate hypothesis: p > 0.14

- The rejection is defined by the significance level ( α = 0.01 ). The rejection region is defined by upper tail of standard normal.

- The Z-critical value that limits the rejection region is defined as:

P ( Z < Z-critical ) = 1 - 0.01 = 0.99

Z-critical = 2.33

- All values over Z-critical are rejected.

- Determine the test statistics by first determining the population standard deviation ( σ ):

- Estimate σ using the given formula:

σ =
`\sqrt{(p*(1-p))/(n) } = \sqrt{(0.14*(1-0.14))/(590) }= 0.01428`

- The Z-test statistics is now evaluated:

Z-test = ( p^ - p ) / σ

Z-test = ( 0.1763 - 0.14 ) / 0.01428

Z-test = 2.542

- The Z-test is compared whether it lies in the list of values from rejection region.

2.542 > 2.33

Z-test > Z-critical

Hence,

Null hypothesis is rejected

- The claim made over the effectiveness of campaign is statistically correct.

Answer 2

a)Null hypothesis:
`p\leq 0.14`

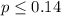
Alternative hypothesis:
`p > 0.14`

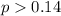
b) For this case we are conducting a right tailed test so then we need to look in the normal standard distribution a quantile that accumulates 0.01 of the are in the left and we got;

`z_(crit) = 2.33`

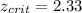
So then the rejection region would be
`(2.33 , \infty)`

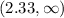
c) The significance level provided
`\alpha=0.01`. The next step would be calculate the p value for this test.

Since is a right tailed test the p value would be:

`p_v =P(z>2.52)=0.006`

And since the p value is lower than the significance level then we can reject the null hypothesis. So then we can conclude that the true proportion of interest is higher than 0.14 at 1% of significance.

Explanation:

Data given and notation

n=590 represent the random sample taken

X=104 represent the drivers were wearing their seat belts

We can estimate the sample proportion like this:

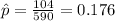`\hat p=(104)/(590)=0.176` estimated proportion of drivers were wearing their seat belts

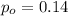`p_o=0.14` is the value that we want to test

`\alpha=0.01` represent the significance level

Confidence=95% or 0.95

z would represent the statistic (variable of interest)

`p_v` represent the p value (variable of interest)

a) System of hypothesis

We need to conduct a hypothesis in order to test the claim that the true proportion of drivers were wearing their seat belts is higher than 0.14 or no, so the system of hypothesis are.:

Null hypothesis:
`p\leq 0.14`

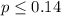
Alternative hypothesis:
`p > 0.14`

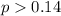
Part b

For this case we are conducting a right tailed test so then we need to look in the normal standard distribution a quantile that accumulates 0.01 of the are in the left and we got;

`z_(crit) = 2.33`

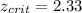
So then the rejection region would be
`(2.33 , \infty)`

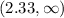
Part c

The statistic is given by:

`z=\frac{\hat p -p_o}{\sqrt{(p_o (1-p_o))/(n)}}` (1)

Calculate the statistic

Since we have all the info requires we can replace in formula (1) like this:

`z=\frac{0.176 -0.14}{\sqrt{(0.14(1-0.14))/(590)}}=2.52`

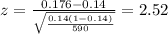
Statistical decision

The significance level provided
`\alpha=0.01`. The next step would be calculate the p value for this test.

Since is a right tailed test the p value would be:

`p_v =P(z>2.52)=0.006`

And since the p value is lower than the significance level then we can reject the null hypothesis. So then we can conclude that the true proportion of interest is higher than 0.14 at 1% of significance.