Answer:
The owner is expected to sell 840 thick crust pizzas.
Explanation:
The Mancini's Pizzeria sells four types of pizza crust:
Thin crust, Thick crust, Stuffed crust and Pan style.
The number of pizzas sold of each type in the past week are:
Thin crust: 291
Thick crust: 234
Stuffed crust: 180
Pan style: 270
_______________
Total: 975
Compute the proportion of each type of pizzas sold.
Proportion of Thin crust

Proportion of Thick crust
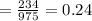
Proportion of Stuffed crust
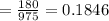
Proportion of Pan style
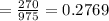
Now let the random variable X be defined as the number of thick crust pizzas sold.
The number of pizzas sold, n = 3500.
The proportion of thick crust pizzas, p = 0.24.
The random variable X follows a Binomial distribution with parameters n = 3500 and p = 0.24.
The expected value of a binomial random variable is:

Compute the expected number of thick crust pizzas sold as follows:

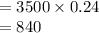
Thus, the owner is expected to sell 840 thick crust pizzas.