Question

There are 4 standard U.S. coins. If a person has one of each. How many different amounts of money can the person give someone using 3 coins?

Answer 1

The different amounts of money can the person give someone using 3 coins are {$0.16, $0.31, $0.36 and $0.40}.

Explanation:

The four standard US coins are:

• Penny (P)
• Nickel (N)
• Dime (D)
• Quarter (Q)

The values of these coins in US dollars are as follows:

• 1 penny = $0.01
• 1 Nickel = $0.05
• 1 dime = $0.10
• 1 quarter = $0.25

Now it is said that a person has one coin of each type.

The person needs to give 3 coins to someone.

The number of ways in which he can select 3 coins from these 4 is:

`{4\choose 3}=(4!)/(3!(4-3)!)=(4* 3!)/(3!* 1!)=4`

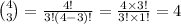
So there are 4 different ways to give 3 coins from 4.

The person can select 3 coins as follows:

{P, N, D}, {P, N, Q}, {P, D, Q} and {N, D, Q}

Compute the total amount of these selections as follows:

{P, N, D} = $0.01 + $0.05 + $0.10 = $0.16

{P, N, Q} = $0.01 + $0.05 + $0.25 = $0.31

{P, D, Q} = $0.01 + $0.10 + $0.25 = $0.36

{N, D, Q} = $0.05+ $0.10 + $0.25 = $0.40

Thus, the different amounts of money can the person give someone using 3 coins are {$0.16, $0.31, $0.36 and $0.40}.