Answer:
a) Spring constant,k = 63.09 N/m
b) Unstretched length = 20.68 m
Step-by-step explanation:
a) Mass of the bungee jumper, M = 60 kg
time for complete oscillation, t = 49 s
Number of oscillations, n = 8
The period of oscillation, T = t/n
T = 49/8
T = 6.125 s
To calculate the spring constant of the bungee cord, use the formula:
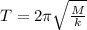
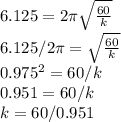
Spring constant,k = 63.09 N/m
b) The extension in the spring is given by the formula:
x = mg / K
x = (60*9.8)/63.09
x = 9.32 m
extension, x = final length - original length
Original length = Final length - extension
Original length = 30 - 9.32
Original length = 20.68m
Unstretched length = 20.68 m