Question

4. Alpha Electronics can purchase a needed service for $90 per unit. The same service can be provided by equipment that costs $100,000 and that will have a salvage value of 0 at the end of 10 years. Annual operating costs for the equipment will be $7,000 per year plus $25 per unit produced. MARR is 12% per year.a) Whats the annual worth if the expected production is 90units/year? 510units/year?

b)Determine the breakeven value for annual production that will return MARR on the investment in the new equipment.

Answer 1

a. $45900 or $37200

b. 380 units

Step-by-step explanation:

Step 1

There are two options available for production. In the first option, the company has to purchase the service at $90 per unit. Thus, the annual cost of the production is 90x. The second option is to purchase the equipment that costs $100,000 with a useful life of 10 years. The operating cost is $7,000 per year plus $25 per unit. The MARR is 12%.

Step 2

First calculate the annual worth of the second option, then calculate the annual cost from both the options and conclude the result.

Step 3

Following is the formula to calculate the annual worth of the second option: AW = P(A/P, i, n) + A

Here,

P is the initial cost of the equipment ($100,000)

A is the annual cost of the equipment (3700+25x)

i is the interest rate (12% or 0.12)

Step 4

Substitute the values in above equation.

AW = $100,000(A/P, 0.12, 10)+ ($7 ,000+25x) = $100,000(0.1770) + $7000 + 25x = S17,700 + $7,000 + 25x

$24,700 + 25x

Thus, the annual cost of the second option is $24,700 + 25x

Step 5

(a) i

Now it is given that the expected production level is 510 units per year. Calculate the annual cost from both the option available.

First Method:

AC =90(x) =90x(510)

AC =S45900

Thus, the annual cost to produce 510 units from the first method is $45,900

(a) ii

Second Method: AC = $24, 700 + 25x = $24.700+ 25(500)

$37,200

Thus, the annual cost to produce 500 units from the second method is $37,200

Conclusion: It is clear from the above calculations that the annual cost of producing 500 units is minimum under second method. Thus, the equipment should be purchased.

(b)

The breakeven point for annual production is that point where the annual cost of both the alternatives are equal.

The calculation is shown below:

`AC_(first) = AC_(second)`

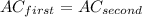
Here,

AC first is the annual cost of production from first method (904

AC second is the annual cost of production from second method ($24,700+25x)

Substitute the values in above equation.

90x = 24,700+25x

65x = 24,700

x = 24,700/65

x = 380

Thus, the annual production that will return MARR on the investment in the new equipment is 380 units.