Answer:
Null Hypothesis,
 :
:
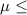 4.75
4.75
Alternate Hypothesis,
 :
:
 > 4.75
> 4.75
Explanation:
We are given that 260 people are randomly chosen at a shopping mall to taste-test a new brand of fruit drink. The results of the survey reveal that the average rating is 5.22 with a standard deviation of 2.31.
The marketing division of the fruit drink distributor is only interested in selling this drink if the true mean rating is more than 4.75.
Let
 = true mean rating.
= true mean rating.
So, Null Hypothesis,
 :
:
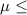 4.75
4.75
Alternate Hypothesis,
 :
:
 > 4.75
> 4.75
Here, null hypothesis states that the true mean rating is less than or equal to 4.75.
On the other hand, alternate hypothesis states that the true mean rating is more than 4.75.
Also, The test statistics that will be used here is One-sample t test statistics as we don't know about population standard deviation;
T.S. =
 ~
~

Hence, the alternative hypothesis for testing whether the fruit drink distributor should sell this drink is
 > 4.75.
> 4.75.