Answer:
The probability value is almost equal to 0. Implying that the proportion of people dying in that particular interval if deaths occurred randomly throughout the year is unusual.
Explanation:
The random variable X can be defined as the number of decedents who died in the three-month period following their birthdays.
A random sample of 747 obituaries published recently in Salt Lake City newspapers revealed that 344 (or 46%) of the decedents died in the three-month period following their birthdays (123).
The probability (p) of anyone dying in any quarter if people die randomly during the year is simply 0.25.
The random variable X follows a Binomial distribution with parameters n = 747 and p = 0.25.
But the sample selected is too large and the probability of success is small.
So a Normal approximation to binomial can be applied to approximate the distribution of p if the following conditions are satisfied:
- np ≥ 10
- n(1 - p) ≥ 10
Check the conditions as follows:
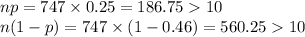
Thus, a Normal approximation to binomial can be applied.
So,
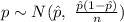 .
.
Compute the probability that 46% or more would die in that particular interval if deaths occurred randomly throughout the year as follows:
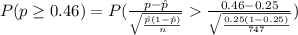
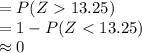
*Use a z table for the probability.
The probability value is almost equal to 0. This probability is very low indicating that the proportion of people dying in that particular interval if deaths occurred randomly throughout the year is unusual.