Question

A 90% confidence interval for the true percentage of college students who like Brussels sprouts is (1.8%, 4.6%). What is the point estimator of the true percentage of college students who like Brussels sprouts?

Answer 1

`\hat p = (Lower+Upper)/(2)`

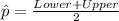
And replacing the info from the problem we have:

`\hat p = (0.018+0.046)/(2)= 0.032`

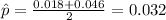
So then the best estimator for the true proportion p is given by
`\hat p = 0.032` or equivalent to 3.2 %

Explanation:

We want to find a confidence interval for a proportion p who represent the parameter of interest.

The confidence interval would be given by this formula:

`\hat p \pm z_(\alpha/2) \sqrt{(\hat p(1-\hat p))/(n)}`

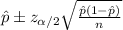
For this case the 90% confidence interval is given by (1.8%=0.018, 4.6%=0.046) after apply the last formula

Since the confidence interval is symmetrical we can estimate the point estimator of the true percentage with this formula:

`\hat p = (Lower+Upper)/(2)`

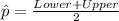
And replacing the info from the problem we have:

`\hat p = (0.018+0.046)/(2)= 0.032`

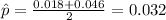
So then the best estimator for the true proportion p is given by
`\hat p = 0.032` or equivalent to 3.2 %