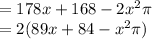Question

Short Response

6. A rectangular soccer field with a length of 5x and a width of 9x has been

marked inside a rectangular field that has a length of 5x + 12 and a width

of 9x + 14.

a. What is the area of the part of the field that is outside the soccer field?

Factor your answer.

b. There is a semicircular fountain in the rectangular field that has a radius of

2x. What is the area of the part of the field that does not include the soccer

field or the fountain? Factor your answer.

Answer 1

(a)2(89x+84)

(b)
`2(89x+84-x^2\pi)`

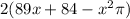
Explanation:

The dimensions of the larger rectangular field are:

• Length=5x + 12; Width = 9x + 14.

The dimensions of the smaller rectangular soccer field are:

• Length=5x; Width = 9x.

(a)Area of the part of the field that is outside the soccer field

=Area of the larger rectangular field - Area of the Soccer Field

=(5x+12)(9x+14)-5x(9x)

=(5x)(9x)+70x+108x+168-5x(9x)

=178x+168

=2(89x+84)

(b)Radius of the Semicircular Fountain =2x

From Part (a),

Area of the larger rectangular field - Area of the Soccer Field=178x+168

Area of the Semicircular Fountain =
`(\pi r^2)/(2) =(\pi (2x)^2)/(2) =(4x^2\pi)/(2) =2x^2\pi`

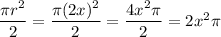
Area of the Field that does not include the soccer field or the fountain.

=Area of the larger rectangular field - Area of the Soccer Field-Area of the Semicircular Fountain

`=178x+168-2x^2\pi\\=2(89x+84-x^2\pi)`