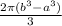Question

A half melon is approximated by the region between two concentric spheres, one of radius a and the other of radius with くa < write a triple integral including in its of integration, giving the volume of the half-melon. Evaluate the integral Enter the exact answer Use φ to represent φ Click here to enter or edit your answer The integral in the appropriate coordinate is ________________

Answer 1

V =
`(2\pi(b^(3) -a^(3)) )/(3)`

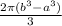
Explanation:

using spherical cordinates :

region is 0≤θ≤2π,0≤ϕ≤π/2,a≤ρ≤b

dV=ρ2sinϕ dρ dϕ dθ

Volume,V=∫[0 to 2π] ∫[0 to π/2] ∫[a to b] dV

=>V=∫[0 to 2π] ∫[0 to π/2] ∫[a to b] ρ2sinϕ dρ dϕ dθ is the integral in appropriate coordinates

=>V=∫[0 to 2π] ∫[0 to π/2] |[a to b] (1/3)ρ3sinϕ dϕ dθ

=>V=∫[0 to 2π] ∫[0 to π/2] (1/3)(b3-a3)sinϕ dϕ dθ

=>V=∫[0 to 2π] |[0 to π/2] (1/3)(b3-a3)(-cosϕ) dθ

=>V=∫[0 to 2π] (1/3)(b3-a3)((-cos(π/2))-(-cos0)) dθ

=>V=∫[0 to 2π] (1/3)(b3-a3)((0)-(-1)) dθ

=>V=∫[0 to 2π] (1/3)(b3-a3)dθ

=>V=|[0 to 2π] (1/3)(b3-a3)θ

=>V= (1/3)(b3-a3)(2π-0)

V =
`(2\pi(b^(3) -a^(3)) )/(3)`