Question

Logbase7(a) * logbaseb(7)

Which of the following are equivalent
A. Log(7)
B. Log(7a)
C. Logbasea(b)
D. Logbaseb(a)

Answer 1

D

Explanation:

log7(a) × logb(7)

Using change of base law, let's convert all of them into lg

lg(a)/lg(7) × lg(7)/lg(b)

lg(a)/lg(b)

Again, using change of base law we can convert this into:

logb(a)

Answer 2

D

Explanation:

We have
`(log_7a)*(log_b7)`. Remember the logarithm properties to turn this into log base 10. For a logarithm
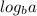`log_ba`, it is equivalent to
`(log(a))/(log(b))`.

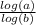So:

`(log_7a)*(log_b7)=(log(a))/(log(7)) *(log(7))/(log(b))`

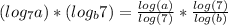
The log(7) cancel out and we're left with:

`(log(a))/(log(b))`

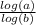
We can now work backwards and turn this into one logarithm. Since before, we had
`log_ba` ⇒
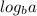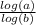`(log(a))/(log(b))`, we now have
`(log(a))/(log(b))` ⇒
`log_ba`.

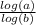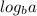Thus, the answer is D.

Hope this helps!