Question

Angle c is inscribed in circle o. ab is diameter of circle o. what is the measure of b

Answer 1

Please consider the attached graph of circle O.

We have been given that angle C is inscribed in circle O. AB is diameter of circle O. We are asked to find the measure of angle B.

We can see that angle C is inscribed angle of diameter AB. We know that an inscribed angle whose endpoints are a diameter is a right angle. So measure of angle C will be 90 degrees.

We also know that all angles of a triangle add up-to 180 degrees, so we can set an equation as:

`\angle A+\angle B+\angle C=180^(\circ)`

`46^(\circ)+\angle B+90^(\circ)=180^(\circ)`

`\angle B+136^(\circ)=180^(\circ)`

`\angle B+136^(\circ)-136^(\circ)=180^(\circ)-136^(\circ)`

`\angle B=44^(\circ)`

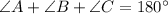
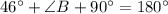
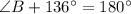
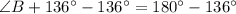
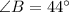
Therefore, the measure of angle B is 44 degrees.