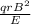Question

By considering the magnetic force in the second region, develop a mathematical expression that relates the mass of the particle to the other variables. Do not include the velocity in your expression. You can use the condition that the particle passed through the region of electric and magnetic fields undeflected to eliminate v from your expression. Your expression will also contain the radius of the circular path. i. Your expression for m should depend on B, E, r, and q

Answer 1

M =
`(qrB^(2) )/(E)`

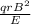
Step-by-step explanation:

considering the magnetic force in the second region derive a mathematical expression that equates the mass of the particle to other variables

In a magnetic field

q = charge, M = mass of particle, E = electric field,B= magnetic field

qvb =
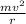`(mv^(2) )/(r)` = therefore m =
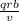`(qrb)/(v)` (equation 1)

note : the particle passes through the region undeflected

therefore : qvb = qE therefore (E = VB)

hence v =
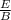`(E)/(B)` ( equation 2 )

insert equation 2 into equation 1

m =
`(qrB^(2) )/(E)`