Answer:
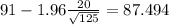
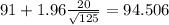
So on this case the 95% confidence interval would be given by (87.494; 94.506). We have 95% of confidence that the true mean is between the limits founded.
Explanation:
Notation
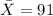 represent the sample mean
represent the sample mean
 population mean (variable of interest)
population mean (variable of interest)
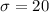 represent the population standard deviation
represent the population standard deviation
n=125 represent the sample size
Confidence interval
The confidence interval for the mean if we know the deviation is given by the following formula:
 (1)
(1)
Since the Confidence is 0.95 or 95%, the value of significance is
 and
and
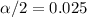 , and we can use excel, a calculator or a table to find the critical value. The excel command would be: "=-NORM.INV(0.025,0,1)".And we see that
, and we can use excel, a calculator or a table to find the critical value. The excel command would be: "=-NORM.INV(0.025,0,1)".And we see that
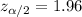
Now we have everything in order to replace into formula (1):
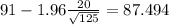
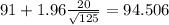
So on this case the 95% confidence interval would be given by (87.494; 94.506). We have 95% of confidence that the true mean is between the limits founded.