Question

The radius of the base of a metallic rod is 2 centimeters, and its height is 15 centimeters. What is the volume of this cylinder?

A) 60π cm³

B) 50π cm³

C) 27π cm³

D) 36π cm³

Answer 1

The correct option is option (A)

The volume of the metallic rod is 60π cm³.

Explanation:

Cylinder:

• It is three dimension shape.
• The lateral surface area is 2πrh, r= radius, h= height

• Total surface area = 2πrh+2πr²,
• Volume = πr²h.

The radius of the base of metallic rod is 2 cm and its height is 15 cm.

Here r= 2 cm, h= 15 cm,
`\pi =\frac{22}7`

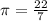
The volume of metallic rod is

=πr²h

=π(2 cm)² (15 cm)

=π(4)(15) cm³

=60π cm³

Answer 2

A) 60π cm³

Explanation:

Given:

The radius of the base of a metallic rod is 2 centimetres, and its height is 15 centimetres.

Question asked:

What is the volume of this cylinder?

Solution:

As we know''

`Volume\ of\ cylinder=\pi r^(2) h`

`=\pi *2*2*15\\ \\ =60\pi`

Therefore, the volume of this cylinder will be
`60\pi\ cm^(3)`