Question

Air of temperature T[infinity] = 27 °C is being used to cool an engine. Consider a single cooling fin at a temperature of Ts = 523 K that can be approximated as a rectangular plate that is 150 cm long by 10 cm high. Air is flowing parallel over both surfaces of the plate. The air flow across the plate at 50 miles per hour may be assumed turbulent throughout. What is the rate of heat removal q in Watts from the cooling fin?

Answer 1

q = 4870.0524 W

Step-by-step explanation:

Given that:

`T_( \infty) = 27^0C = (27+273)K = 300 K`

`T_s = 523K`

`T_f = (T _\infty- T_s )/(2)`

`T_f = (300-523)/(2)`

`T_f =412 K`

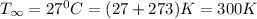
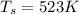
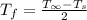
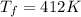
From Table A4; Thermophysical Properties of Gases at Atmospheric Temperature; Properties of air at film Temperature are as follows:

density
`\rho = 0.8478 \ kg/m^3`

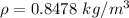
kinematic viscosity (v) =
`27.845*10^(-6) \ m^2/s`

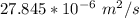
thermal conductivity K =
`34.64 *10^(-3 ) \ W/m.K`

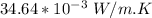
Prandtl number (Pr) = 0.689

Given that :

the length of the plate is = 150 cm = 1.5 m

the width of the plate = 10 cm = 0.1 m

Then the Area = W×L = 1.5 × 0.10 = 0.15 m²

Air flow velocity
`(u_(\infty ) )= 50 \ mph = 22.35 m/s`

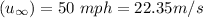
The Reynolds number
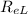`R_(eL)` is calculated by using the formula :

`R_(eL) = (\mu_(\infty)L)/(v)`

`R_(eL) = (22.35*1.5)/(27.845*10^(-6))`

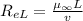
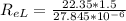
`R_(eL) = 1.2*10^6` turbulent flow.

Thus, we can say that the turbulent flow is throughout the entire plate. Therefore, the appropriate correlation is addressed via the Nusselt number;

`N \bar {u} _L = (0.037R_(eL) -A ) Pr ^(1/3)`

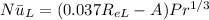
where;

`A = 0.037 \ Re ^(4/5)_(x,e) - 0.664 \ Re ^(1/2)_(x,e)`

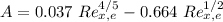
and
`Re_(x.e) = 5*10^5`

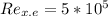
Then;

`A = 0.037 \ *5*10^5 ^((4/5))- 0.664 \* 5*10^5 ^((1/2))`

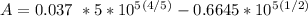
A = 871

Now;

`N \bar {u} _L = (0.037*1.2*10^6-871 ) *0.689 ^(1/3)`

`N \bar {u} _L = 3152.24`

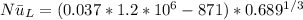
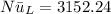
We can now determine the convention coefficient since the
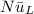`N \bar {u} _L` is known. By using the equation;

`\bar {h} = \frac{N \bar {u} _L *k}{L}`

`\bar {h} = (3152.24*34.64*10^(-3))/(1.5)`

`\bar {h} =72.796 \ W/m^2 .K`

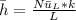
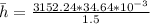
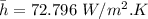
Finally the rate of heat removal q from both cooling surface of the plate (fin) is calculated as:

`q= 2*A * \bar {h} (T_s - T _ {\infty})`

`q= 2*0.15 * 72.796 (523 - 300)`

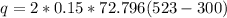
q = 4870.0524 W