Question

Use the data to find the interquartile range. [Note: Type your answers as numbers. Do not round.] Movie Ticket Fees at a Local Theater $10.25 $9.75 $8.75 $12.75 $11.00 $6.50 . Range: $ ___a0 Interquartile Range: $ ___a1

Answer 1

The range is 6.25 and the interquartile range is 2.25

Explanation:

Answer 2

The Inter quartile range is $2.25.

Explanation:

The Inter quartile range (IQR) is a quantity which tells amount of data contained between the first quartile (Q₁) and third quartile (Q₃).

The IQR can be computed using the formula:

`IQR=Q_(3)-Q_(1)`

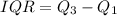
The first quartile (Q₁) is well defined as the mid-value between the minimum value and the median of the data set. Or the mid-value of the first half of the data set is the first quartile.

The third quartile (Q₃) is the mid-value amid the median and the maximum value of the data set. Or the mid-value of the second half of the data set is the Third quartile.

The data set in arranged order is:

S = {$6.50, $8.75, $9.75, $10.25, $11.00, $12.75}

The first half of the data set is:

S₁ = {$6.50, $8.75, $9.75}

The mid-value is $8.75.

The first quartile is, Q₁ = $8.75.

The second half of the data set is:

S₁ = {$10.25, $11.00, $12.75}

The mid-value is $11.00.

The first quartile is, Q₃ = $11.00.

Compute the Inter quartile range as follows:

`IQR=Q_(3)-Q_(1)`

`=11.00-8.75\\=2.25`

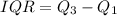
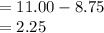
Thus, the Inter quartile range is $2.25.