Question

Water at 20 bar, 400oC enters a turbine operating at steady state and exits at 1.5 bar. Neglect heat transfer, kinetic energy and potential energy effects. Someone states that the vapor quality at the turbine exit is 98%. (a) (35%) Find the entropy generation based on this statement. (b) (5%) Is this statement possible?

Answer 1

a)
`s_(gen) = -0.0219\,(kJ)/(kg\cdot K)`, b) No.

Step-by-step explanation:

The turbine is modelled after the Second Law of Thermodynamics, which states:

`s_(in) - s_(out) + s_(gen) = 0`

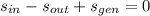
The entropy generation per unit mass is:

`s_(gen) = s_(out) - s_(in)`

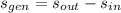
The specific entropy for steam at entrance and exits are obtained from property tables:

Inlet (Superheated Steam)

`s_(in) = 7.1292\,(kJ)/(kg\cdot K)`

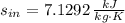
Outlet (Liquid-Vapor Mixture)

`s_(out) = 7.1073\,(kJ)/(kg\cdot K)`

`s_(gen) = 7.1073\,(kJ)/(kg\cdot K) - 7.1292\,\frac {kJ}{kg\cdot K}`

`s_(gen) = -0.0219\,(kJ)/(kg\cdot K)`

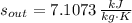
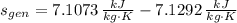
b) It is not possible, as it contradicts the Kelvin-Planck and Claussius Statements, of which is inferred that entropy generation can only be zero or positive.