Question

Points A and B lie on a circle with radius 1, and arc ⌢ AB has a length of π3. What fraction of the circumference of the circle is the length of arc ⌢ AB?

Answer 1

1/6

Step-by-step explanation:

The circumference of a circle is denoted by:
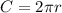`C=2\pi r`, where r is the radius. Here, r = 1, so plug this in:

`C=2\pi r`

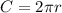
`C=2\pi *1=2\pi` units

Now, we know that arc AB has a length of
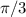`\pi /3` and we want to find the fraction of the circumference this is. So, divide
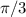`\pi /3` by
`2\pi`:

`(\pi /3)/(2\pi )=(\pi )/(3*2\pi )=(\pi )/(6\pi ) =1/6`

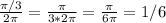
Thus, the fraction is 1/6.

Hope this helps!

Answer 2

arc AB =1/6 circumference

Step-by-step explanation:

The circumference of a circle with radius r = 1

has a length C 1 =2r π =2 π

An arc length of π/3

represents π/3/2π = 1/3/2 +1/ 6 of this circumference

Sorry all those //// are those fraction line but i couldn't find them in these symbols hope this helpful.I get it.