Answer:
a) the number of bacteria in the dish after an additional hour has passed = 8
b) the carrying capacity of the dish based on the developed logistic equation. = 9
Explanation:
Given that ;
The Logistic growth model is :
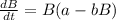
Solving the above equation ; we have a MODEL EQUATION;
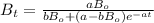
in which:
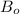 represents the initial population of the bateria.
represents the initial population of the bateria.
We are given that the number of the bacteria in the Petri dish was initially determined to be 1 , then ;

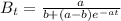
After an hour ; we were told that the number of the bacteria increased to 3 ; So:
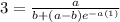
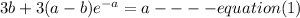
Similarly after (2) hours; the number of the bacteria increased to 6; then
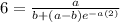
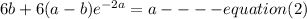
So;
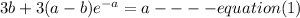
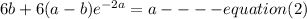
Solving for a and b from the above two eqautions: Then,
a = 1.386
b = 0.154
Substituting the value of a and b into our MODEL EQUATION; we have
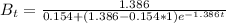

a) Determine the number of bacteria in the dish after an additional hour has passed.
i.e at t = 3
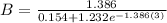
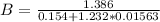


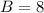
b) Determine the carrying capacity of the dish based on the developed logistic equation.
The carrying capacity B can be expressed as :
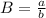
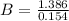
B =9