Question

In 1982 Abby’s mother scored at the 93rd percentile in the math SAT exam. In 1982 the mean score was 503 and the variance of the scores was 9604. In 2008 Abby took the math SAT and got the same numerical score as her mother had received 26 years before. In 2008 the mean score was 521 and the variance of the scores was 10,201. Math SAT scores are normally distributed.

Calculuate the percentile for Abby's score.

Answer 1

The percentle for Abby's score was the 89.62nd percentile.

Explanation:

Problems of normally distributed samples are solved using the z-score formula.

In a set with mean
`\mu` and standard deviation(which is the square root of the variance)
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

Abby's mom score:

93rd percentile in the math SAT exam. In 1982 the mean score was 503 and the variance of the scores was 9604.

93rd percentile. X when Z has a pvalue of 0.93. So X when Z = 1.476.

`\mu = 503, \sigma = √(9604) = 98`

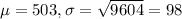
So

`Z = (X - \mu)/(\sigma)`

`1.476 = (X - 503)/(98)`

`X - 503 = 1.476*98`

`X = 648`

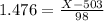
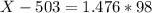
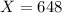
Abby's score

She scored 648.

`\mu = 521 \sigma = √(10201) = 101`

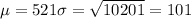
So

`Z = (X - \mu)/(\sigma)`

`Z = (648 - 521)/(101)`

`Z = 1.26`

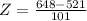
`Z = 1.26` has a pvalue of 0.8962.

The percentle for Abby's score was the 89.62nd percentile.