Question

in order to qualify for a police academy, applicants are given a test of physical fitness. The scores are normally distributed with a mean of 60 and a standard deviation of 12. If only the top 20% of the applicants are selected find the cutoff score. Round value calculations to 2 decimal places and final answer to the nearest whole number

Answer 1

The cutoff score is 71.

Explanation:

We are given that the scores are normally distributed with a mean of 60 and a standard deviation of 12.

If only the top 20% of the applicants are selected we have to find the cut off score.

Let X = sample mean daily precipitation

So, X ~ Normal(
`\mu=60,\sigma^(2) =12^(2)`)

The z score probability distribution for normal distribution is given by;

Z =
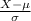`( X-\mu)/(\sigma ) }` ~ N(0,1)

where,
`\mu` = population mean score = 60

`\sigma` = standard deviation = 12

Now, we have to find the cut off score at which the top 20% of the applicants are selected, that is;

P(X
`\geq` x) = 0.20 {where x is the required cut off score}

P(
`( X-\mu)/(\sigma ) }`
`\geq`
`(x-60)/(12 ) }` ) = 0.20

P(Z
`\geq`
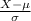
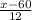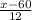`(x-60)/(12 ) }` ) = 0.20

Now, in the z table the critical value of x that represents the top 20% of the area is given as 0.8416, i.e;

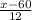`(x-60)/(12 ) }` = 0.8416

`{x-60}}= 0.8416 * 12`

x = 60 + 10.09 = 70.09 ≈ 71

Hence, the cutoff score is 71.