Question

In ΔOPQ, the measure of ∠Q=90°, QO = 5.9 feet, and OP = 8 feet. Find the measure of ∠P to the nearest tenth of a degree.

Answer 1

47.5°

Explanation:

\sin P =

hypotenuse

opposite

= 5.9/8

sin P= 5.9/8

P=sin -1

(5.9/8)

P=47.5189 ≈ 47.5°

​

Answer 2

Measure of
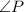`\angle P` to nearest tenth of a degree is
`47.5^(\circ)`.

Explanation:

Diagram of given scenario is shown below.

Given that,

A right angle triangle
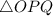`\triangle OPQ`. Base of triangle is
`QP`.

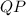Perpendicular side of triangle is
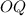`OQ` and Hypotenuse side of triangle is
`OP`.

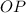In
`\triangle OPQ`,
`\angle Q=90^(\circ)`,
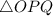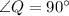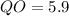`QO=5.9` and
`OP=8`.

Now, Using Trigonometry ratio:

`Sin\theta=(Perpendicular)/(Hypoteneuse)`

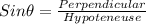
So, Substituting the values of perpendicular and hypotenuse we get:

`Sin\angle P = (OQ)/(OP) =(5.9)/(8)`

`Sin\angle P = 0.7375` ⇒
`\angle P= Sin^(-1)(0.7375)=47.51888^(\circ)`

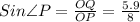
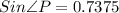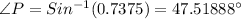
Therefore, Measure of
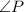`\angle P` to nearest tenth of a degree is
`47.5^(\circ)`.