Question

J Corp. common stock is priced at $36.50 per share. The company just paid its $0.50 quarterly dividend. Interest rates are 6.0%. A $35.00 strike European call, maturing in 6 months, sells for $3.20. What is the price of a 6-month, $35.00 strike put option

Answer 1

Step-by-step explanation:

The time (T) = 6 months = 6/12 years = 0.5 years

Interest rate (r) = 6% = 0.06

The stock is priced [S(0)] = $36.50

The price the stock sells at 6 months (
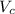`V_c`) = $3.20

European call (K) = $35

The price (P) is given by:

`P=V_c+K.e^(-rT)-S(0)+Dividends\\But, Dividends = 0.5*e^(-0.25*0.06)+ 0.5*e^(-0.5*0.06)\\Therefore, P=V_c+K.e^(-rT)-S(0)+0.5*e^(-0.25*0.06)+ 0.5*e^(-0.5*0.06)\\Substituting:\\P=3.2+35*e^(-0.06*0.5)-36.5+0.5*e^(-0.25*0.06)+ 0.5*e^(-0.5*0.06)\\P=3.2+33.9656-36.5+0.4926+0.4852\\P=1.64`

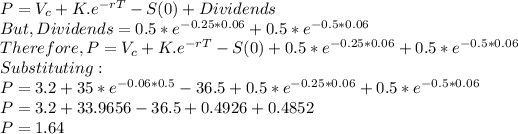
The price of a 6-month, $35.00 strike put option is $1.65