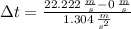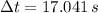Answer:
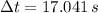
Step-by-step explanation:
The figure of the problem is included below as attachment. The equations of equilibrium are presented below:

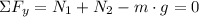
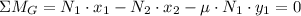
The system of equations are:
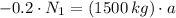
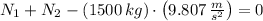
![N_(1) \cdot [(0.75\,m)-0.2\cdot (0.35\,m)] - N_(2)\cdot (1.25\,m) = 0](https://img.qammunity.org/2021/formulas/physics/college/2gvrik9jmolggt9oztltoldq1ai876yqqc.png)
The solution of the system is:
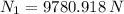 ,
,
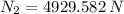 and
and
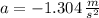
The shortest time to reach a speed of 80 km/h is: