Let x represent amount invested in the higher-yielding account.
We have been given that a man puts twice as much in the lower-yielding account because it is less risky. So amount invested in the lower-yielding account would be
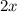 .
.
We are also told that his annual interest is $6600 dollars. We know that annual interest for one year will be principal amount times interest rate.
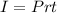 , where,
, where,
I = Amount of interest,
P = Principal amount,
r = Annual interest rate in decimal form,
t = Time in years.
We are told that interest rates are 6% and 10%.
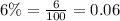

Amount of interest earned from lower-yielding account:
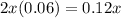 .
.
Amount of interest earned from higher-yielding account:
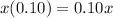 .
.
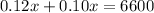
Let us solve for x.
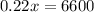
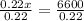
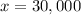
Therefore, the man invested $30,000 at 10%.
Amount invested in the lower-yielding account would be
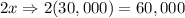 .
.
Therefore, the man invested $60,000 at 6%.