Answer : The enthalpy of neutralization is, -55.8 KJ/mole
Explanation :
First we have to calculate the moles of
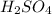 and KOH.
and KOH.
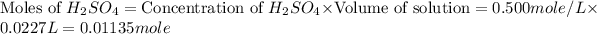

Now we have to calculate the limiting reagent.
The balanced chemical reaction will be,
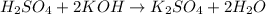
From the balanced reaction we conclude that,
As, 1 mole of
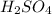 react to give 2 moles of
react to give 2 moles of
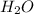
So, 0.01135 mole of
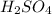 react to give
react to give
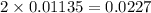 moles of
moles of
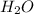
and,
As, 2 moles of
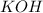 react to give 2 moles of
react to give 2 moles of
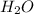
As, 0.0227 moles of
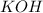 react to give 0.0227 moles of
react to give 0.0227 moles of
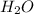
From this we conclude that they can form the same amount of product. This means that both will consume completely in the reaction.
Thus, the number of neutralized moles = 0.0227 mole
Now we have to calculate the mass of water.
As we know that the density of water is 1 g/ml. So, the mass of water will be:
The volume of water =
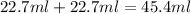
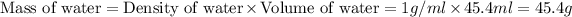
Now we have to calculate the heat absorbed during the reaction.
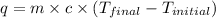
where,
q = heat absorbed = ?
 = specific heat of water =
= specific heat of water =
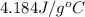
m = mass of water = 45.4 g
 = final temperature of water =
= final temperature of water =
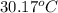
 = initial temperature of water =
= initial temperature of water =
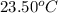
Now put all the given values in the above formula, we get:
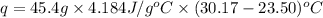

Now we have to calculate the enthalpy of neutralization.
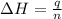
where,
 = enthalpy of neutralization = ?
= enthalpy of neutralization = ?
q = heat released = -1.267 KJ
n = number of moles used in neutralization = 0.0227 mole
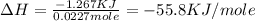
The negative sign indicate the heat released during the reaction.
Therefore, the enthalpy of neutralization is, -55.8 KJ/mole