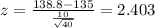Question

The mean yield of corn in the United States is about 135 bushels per acre. A survey of 40 farmers this year gives a sample mean yield of 138.8 bushels per acre. We want to know whether this is good evidence that the national mean this year is not 135 bushels per acre. Assume that the standard deviation of the population is 10 bushels per acre.

Report the value of the test statistic z :

Answer 1

`z = (138.8-135)/((10)/(√(40)))= 2.403`

Explanation:

Data given and notation

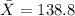`\bar X=138.8` represent the sample mean

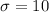`\sigma = 10` represent the population standard deviation

`n=40` sample size

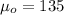`\mu_o =135` represent the value that we want to test

t would represent the statistic (variable of interest)

`p_v` represent the p value for the test (variable of interest)

Solution to the problem

We need to conduct a hypothesis in order to check if the true mean for the bushlels per acre is not 135, the system of hypothesis would be:

Null hypothesis:
`\mu = 135`

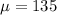
Alternative hypothesis:
`\mu \\eq 135`

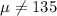
And the z statistic is given by:

`z= (\bar X -\mu)/((\sigma)/(√(n)))`

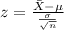
And replacing we got:

`z = (138.8-135)/((10)/(√(40)))= 2.403`