Question

Research at the University of Toledo indicates that 50 percent ofthe students change their major area of study after their firstyear in a program. A random sample of 100 students in the Collegeof Business revealed that 48 had changed their major area of studyafter their first year of the program.Has there been a significant decrease in the proportion of studentswho change their major after the first year in this program? Testat the .05 level of significance.(a) What is the decisionrule? (Round youranswer to 3 decimal places.)(b) The value ofthe test statistic is . (Roundyour answer to 2 decimal places.)(c) What is yourdecision regarding ?

Answer 1

a) The decision rule is: if the P-value is below the level of significance, the null hypothesis is rejected.

b) The value of the test statistic is z=-0.30.

c) There is not enough evidence to support the claim that there has been a significant decrease in the proportion of students who change their major after the first year in this program.

Explanation:

This is a hypothesis test for a proportion.

The claim is that there has been a significant decrease in the proportion of students who change their major after the first year in this program.

Then, the null and alternative hypothesis are:

`H_0: \pi=0.5\\\\H_a:\pi< 0.5`

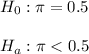
The significance level is 0.05.

The sample has a size n=100.

The sample proportion is p=0.48.

`p=X/n=48/100=0.48`

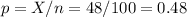
The standard error of the proportion is:

`\sigma_p=\sqrt{(\pi(1-\pi))/(n)}=\sqrt{(0.5*0.5)/(100)}\\\\\\ \sigma_p=√(0.0025)=0.05`

Then, we can calculate the z-statistic as:

`z=(p-\pi-0.5/n)/(\sigma_p)=(0.48-0.5+0.5/100)/(0.05)=(-0.015)/(0.05)=-0.3`

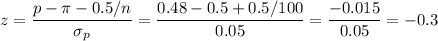
This test is a left-tailed test, so the P-value for this test is calculated as:

`P-value=P(z<-0.3)=0.3821`

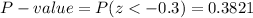
As the P-value (0.3821) is bigger than the significance level (0.05), so the effect is not significant.

The null hypothesis failed to be rejected.

There is not enough evidence to support the claim that there has been a significant decrease in the proportion of students who change their major after the first year in this program.