Question

Forty-six percent of a population possesses a particular characteristic. Random samples are taken from this population. Determine the probability of each of the following occurrences. (Round all z values to 2 decimal places. Round all intermediate calculations and answers to 4 decimal places.)

a. The sample size is 60 and the sample proportion is between .41 and.53.
b. The sample size is 458 and the sample proportion is less than 40
c. The sample size is 1350 and the sample proportion is greater than.49

Answer 1

(a) The value of P (0.41 <
`\hat p` < 0.53) is 0.6444.

(b) The value of P (
`\hat p` < 0.40) is 0.0049.

(c) The value of P (
`\hat p` > 0.49) is 0.9826.

Explanation:

According to the Central limit theorem, if from an unknown population large samples of sizes n > 30, are selected and the sample proportion for each sample is computed then the sampling distribution of sample proportion follows a Normal distribution.

The mean of this sampling distribution of sample proportion is:

`\mu_(\hat p)=p`

The standard deviation of this sampling distribution of sample proportion is:

`\sigma_(\hat p)=\sqrt{(p(1-p))/(n)}`

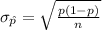
Given that: p = 0.46.

(a)

The sample size is n = 60 > 30. So the central limit theorem can be applied to approximate the sampling distribution of sample proportion by a Normal distribution.

Compute the value of P (0.41 <
`\hat p` < 0.53) as follows:

`P(0.41<\hat p<0.53)=P(\frac{0.41-0.46}{\sqrt{(0.46(1-0.46))/(60)}}<(\hat p-\mu_(\hat p))/(\sigma_(\hat p))< \frac{0.53-0.46}{\sqrt{(0.46(1-0.46))/(60)}})`

`=P(-0.78<Z<1.09)\\=P(Z<1.09)-P(Z<-0.78)\\=0.86214-0.21770\\=0.64444\\\approx0.6444`

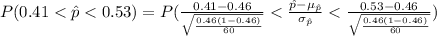
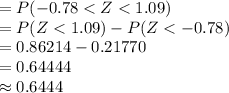
Thus, the value of P (0.41 <
`\hat p` < 0.53) is 0.6444.

(b)

The sample size is n = 458 > 30. So the central limit theorem can be applied to approximate the sampling distribution of sample proportion by a Normal distribution.

Compute the value of P (
`\hat p` < 0.40) as follows:

`P(\hat p<0.40)=P((\hat p-\mu_(\hat p))/(\sigma_(\hat p))<\frac{0.40-0.46}{\sqrt{(0.46(1-0.46))/(458)}})`

`=P(Z<-2.58)\\=0.00494\\\approx 0.0049`

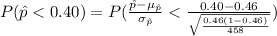
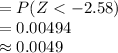
Thus, the value of P (
`\hat p` < 0.40) is 0.0049.

(c)

The sample size is n = 1350 > 30. So the central limit theorem can be applied to approximate the sampling distribution of sample proportion by a Normal distribution.

Compute the value of P (
`\hat p` > 0.49) as follows:

`P(\hat p>0.49)=P((\hat p-\mu_(\hat p))/(\sigma_(\hat p))>\frac{0.49-0.46}{\sqrt{(0.46(1-0.46))/(1350)}})`

`=P(Z<2.11)\\=0.98257\\\approx 0.9826`

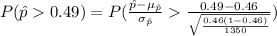
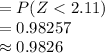
Thus, the value of P (
`\hat p` > 0.49) is 0.9826.

*Use a z-table for all the probability.