Answer:
The fair price for the large container is $12
Explanation:
In this question, we are tasked with calculating what would be the fair price for the large cylinder given the price for the small cylinder.
To calculate this, what we need to do firstly is to estimate the the volumes of both cylinders.
Mathematically, the volume of a cylinder can be calculated using the formula; V = π ×
 ×h
×h
For the small cylinder, r = 3 inches and h = 10 inches
Thus
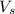 = π ×
= π ×
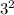 × 10 = 90π
× 10 = 90π
For the large cylinder, r = 6 inches and h = 10 inches
Thus
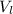 = π ×
= π ×
 × 10 = 360π
× 10 = 360π
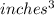
Now let us find the ratio of their volumes = 360π/90π = 4
Since the ratio of their volumes is 4, it can be fair to say that the large cylinder should cost 4 times the price of the small cylinder = 4 × $3 = $12