Question

If the current and voltage of an electrical device (in the passive reference configuration) are v \left (t \ right) v (t) = 6 \sin\left(100\pi t\right)sin(100πt) V and i\left(t\right)i(t) = 6 \sin\left (100 \ pi t \ right) sin (100πt) + 9 \cos \left (100\pi t\right) cos (100πt) A. Then the average power delivered to the device (computed to one decimal place) is given by:___________.

Answer 1

18W

Step-by-step explanation:

To find the average power of the electrical device you use the following formula:

`P(t)=(1)/(T)\int_0^(T)i(t)v(t)dt\\\\i(t)=6sin(100\pi t)+9cos(100\pi t)\\\\v(t)=6sin(100\pi t)`

T: period of the oscillation

`T=(2\pi)/(\omega)=(2\pi)/(100\pi)=(1)/(50)s`

i(t): current

v(t): voltage

By replacing i and v in the integral and solve it you obtain:

The solution of the integral is attached below:

By replacing the value of the integral (9/25) you obtain:

`P=(1)/(1/50)(9)/(25)=18W`

hence, the power of the device is 18W

Answer 2

The average power is 18 W

Step-by-step explanation:

Solution:-

If the current and voltage of an electrical device (in the passive reference configuration) are:

v(t) = 6 sin(100πt) V

i(t) = 6 sin(100πt) + 9 cos(100πt) Amp

- First we will find the harmonic form of the current function i(t):

a sin ( wt ) + b cos ( wt ) ≡ R*sin ( wt + α )

Where,

R = √( a^2 + b^2 )

α = arctan ( b / a )

- Transforming the function i (t) into the harmonic form:

a = 6 , b = 9

R = √( a^2 + b^2 ) = √( 6^2 + 9^2 ) = √( 36 + 81 )

R = √117

α = arctan ( b / a ) = arctan ( 9 / 6 )

α = 0.98279 rads

Hence,

i(t) = 6 sin(100πt) + 9 cos(100πt) ≡ √117*sin (100πt + 0.98279 )

- The average power is given by the following formula:

`P_a_v_g = (V_m*I_m)/(2)*cos ( \beta )`

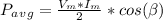
Where,

Vm: The mean voltage

Im : The mean current

β : The phase difference

- Using the given functions we have:

Vm = 6 V , Im = R = √117 Amps , β = 0.98279

`P_a_v_g = (6*√(117) )/(2)*cos ( 0.98279 )\\\\P_a_v_g = 18 W`

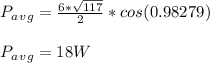
Answer: The average power is 18 W.