Question

From a group of 6 juniors and 5 sophomores a committee of 3 juniors and 3 sophomores is to be selected. one junior is to receive a 12 month term on the committee, one junior is to receive a 9 month term, one junior is to receive a 6 month term and all sophomores are to receive 4 moth terms. How many different committees are possible?

Answer 1

The number of different committees that are possible is 200.

Explanation:

Combinations is a mathematical procedure to determine the number of ways to select k items from n distinct items.

`{n\choose k}=(n!)/(k!(n-k)!)`

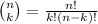
The group consists of 6 juniors and 5 sophomores.

The committee to be formed must consist of 3 juniors and 3 sophomores.

Compute the number of ways to select 3 juniors from 6 as follows:

`n (3\ juniors)={6\choose 3}=(6!)/(3!(6-3)!)=(6!)/(3!*3!)=(6* 5* 4*3!)/(3!* 3!)=20`

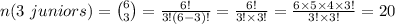
Compute the number of ways to select 3 sophomores from 5 as follows:

`n (3\ sophomores)={5\choose 3}=(5!)/(3!(5-3)!)=(5!)/(3!*2!)=(5* 4*3!)/(3!* 2!)=10`

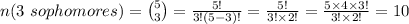
Compute the total number of different committees possible as follows:

Total number of committees possible = n (3 juniors) × n (3 sophomores)

`={6\choose 3}* {5\choose 3}\\=20* 10\\=200`

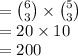
Thus, the number of different committees that are possible is 200.