Question

My favorite pizza restaurant advertises that their average delivery time is 20 minutes. It always feels like it takes forever for my pizza to arrive, so I don't believe this is true. I start recording how long it takes for my pizza order to be delivered and I ask a few of my friends to help out too). Of the 50 pizza deliveries we record, the average delivery time is 20.7 minutes with a standard deviation of 2.1 minutes. Conduct an appropriate hypothesis test at the a = 0.05 level to determine if the advertised delivery time is overly optimistic. Population parameter(s): Sample statistics: Hypotheses: Test Statistic: p-value: Reject H? Conclusion (in context of the problem): In the pizza problem above, you should have found that the difference between the advertised average delivery time and the actual average delivery time was statistically significant. Is this difference practically significant? Explain. Make a 99% confidence interval for the average delivery time. Based on this interval, would we reject the null hypothesis? Does this agree with the hypothesis test you performed above? Why or why not? Explain.

Answer 1

Population parameter(s): mean μ=20

Sample statistics: M=20.7, s=2.1

Hypotheses:

`H_0: \mu=20\\\\H_a:\mu> 20`

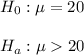
Test Statistic: t=2.357

p-value: 0.011

Reject H? YES

Conclusion (in context of the problem): There is enough evidence to support the claim that the advertised delivery time is overly optimistic and it is larger than 20 minutes.

Is this difference practically significant? No, the difference as the sample mean delivery time is under one minute of difference from the advertised time. Although it is significantly from the statistical point of view, the difference is under 5% of the advertised delivery time.

The 99% confidence interval for the mean is (19.904, 21.496).

With this level of confidence, the null hypothesis failed to be rejected, as t=20 is a possible value for the true mean (is included in the confidence interval).

Explanation:

This is a hypothesis test for the population mean.

The claim is that the advertised delivery time is overly optimistic and it is larger than 20 minutes.

Then, the null and alternative hypothesis are:

`H_0: \mu=20\\\\H_a:\mu> 20`

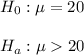
The significance level is 0.05.

The sample has a size n=50.

The sample mean is M=20.7.

As the standard deviation of the population is not known, we estimate it with the sample standard deviation, that has a value of s=2.1.

The estimated standard error of the mean is computed using the formula:

`s_M=(s)/(√(n))=(2.1)/(√(50))=0.297`

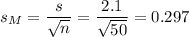
Then, we can calculate the t-statistic as:

`t=(M-\mu)/(s/√(n))=(20.7-20)/(0.297)=(0.7)/(0.297)=2.357`

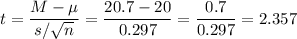
The degrees of freedom for this sample size are:

`df=n-1=50-1=49`

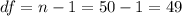
This test is a right-tailed test, with 49 degrees of freedom and t=2.357, so the P-value for this test is calculated as (using a t-table):

`P-value=P(t>2.357)=0.011`

As the P-value (0.011) is smaller than the significance level (0.05), the effect is significant.

The null hypothesis is rejected.

There is enough evidence to support the claim that the advertised delivery time is overly optimistic and it is larger than 20 minutes.

2. We have to calculate a 99% confidence interval for the mean.

The sample mean is M=20.7.

The sample size is N=50.

The t-value for a 99% confidence interval is t=2.68.

The margin of error (MOE) can be calculated as:

`MOE=t\cdot s_M=2.68 \cdot 0.297=0.796`

Then, the lower and upper bounds of the confidence interval are:

`LL=M-t \cdot s_M = 20.7-0.796=19.904\\\\UL=M+t \cdot s_M = 20.7+0.796=21.496`

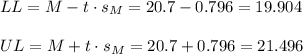
The 99% confidence interval for the mean is (19.904, 21.496).

With this level of confidence, the null hypothesis failed to be rejected, as t=20 is a possible value for the true mean (is included in the confidence interval).