Question

In the diagram shown of circle A, segments UV and UT are congruent. If

mVST  220 , then determine the measure of VSU . Show how you
arrived at your answer.

Answer 1

The measure of angle VSU is 35°.

Explanation:

We know that the arc VST is 220°.

This means arc VUT is 140°, because it's the difference between the total arc 360° and 220°.

Also, we know that segments UV and UT are congruent, that means their arcs are also congruent. So, the measure of arc VUT can be equally divided, that means arc VU is 70°.

Now, the inscribed angle theorem states that the angle formed by two intersecting chords is half of its subtended arc.

`\angle VSU = (1)/(2) arc(VU)=(1)/(2)(70\°) \\ \angle VSU = 35\°`

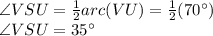
Therefore, the measure of angle VSU is 35°.