Question

As reported on the package of seeds, the mean time until maturity of a certain variety of tomato plant is 70 days. It is also known that the maturity of this variety of tomato follows a normal distributed with standard deviation σ = 2.4. A researcher believes that it will indeed take more time in a given condition. To test his belief, he selects a sample of 16 plants of this variety under the maintained condition and measure the time until maturity. The sample yields

Answer 1

`t=(72-70)/((2.4)/(√(36)))=5`

`p_v =P(z>5)=0.00000`

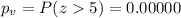
The best option would be:

z=5 and p-value 0.000000

Explanation:

Assuming this complete question: "A researcher believes that it will indeed take more time in a given condition. To test his belief, he selects a sample of 36 plants of this variety under the given condition and measure the time until maturity. The sample mean is found to be 72 days. The value of the test statistic and p-value for testing H0: μ=70, Ha: μ>70 are:

z=5 and p-value 0.000000

z=3 and p-value=0.99865

z=2 and p-value=0.02275"

Data given and notation

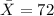`\bar X=72` represent the sample mean

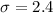`\sigma=2.4` represent the population standard deviation

`n=36` sample size

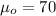`\mu_o =70` represent the value that we want to test

z would represent the statistic (variable of interest)

`p_v` represent the p value for the test (variable of interest)

State the null and alternative hypotheses.

We need to conduct a hypothesis in order to check if the true mean is higher than 70 days, the system of hypothesis would be:

Null hypothesis:
`\mu \leq 70`

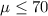
Alternative hypothesis:
`\mu > 70`

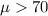
The statistic is given by:

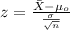`z=(\bar X-\mu_o)/((\sigma)/(√(n)))` (1)

Calculate the statistic

We can replace in formula (1) the info given like this:

`t=(72-70)/((2.4)/(√(36)))=5`

P-value

Since is a one side test the p value would be:

`p_v =P(z>5)=0.00000`

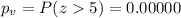
The best option would be:

z=5 and p-value 0.000000