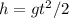Answer:
Contact and tension force: 3mg
Friction force: mg
Distance the hanging block would drop in a time t:
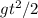
Step-by-step explanation:
The gravity force on the hanging block would be:
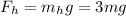
Which is the contact force that directly affect the 2 stacked-block system, which would have an acceleration of:
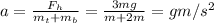
So the friction force that pulls the top block must be
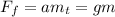
Since the pulley has negligible mass and radius, the tension force would be the same as contact force, which is 3mg
Now that the system moves with an acceleration of g, then after t (seconds), the hanging block would have dropped by a distance of