Answer:
a)
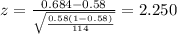
b)
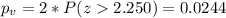
If we compare the p value and the significance level given we see that
 we have enough evidence to reject the null hypothesis at 5% of significance.
we have enough evidence to reject the null hypothesis at 5% of significance.
Explanation:
Data given and notation
n=114 represent the random sample taken
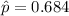 estimated proportion of people that their approval rating might have changed
estimated proportion of people that their approval rating might have changed
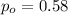 is the value that we want to test
is the value that we want to test
 represent the significance level
represent the significance level
Confidence=95% or 0.95
z would represent the statistic (variable of interest)
 represent the p value (variable of interest)
represent the p value (variable of interest)
Hypothesis
We need to conduct a hypothesis in order to test the claim that true proportion of people that their approval rating might have changed is 0.58 or no.:
Null hypothesis:
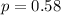
Alternative hypothesis:
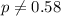
Part a
 (1)
(1)
Calculate the statistic
Since we have all the info requires we can replace in formula (1) like this:
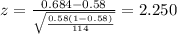
Part b: Statistical decision
The significance level provided
 . The next step would be calculate the p value for this test.
. The next step would be calculate the p value for this test.
Since is a bilateral test the p value would be:
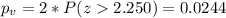
If we compare the p value and the significance level given we see that
 we have enough evidence to reject the null hypothesis at 5% of significance.
we have enough evidence to reject the null hypothesis at 5% of significance.