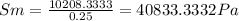Question

A block of gelatin is 120mm by 120mm by 40mm whrn unstressed. A force of 49N is applied tangentially to the upper surface causing a 10mm displacement relative to the lower surface. The block is placed such that 120mm by 120mm comes on the lower and upper surface, find the shear modulus, shearing stress, shearing strain​

Answer 1

The shearing stress is 10208.3333 Pa

The shearing strain is 0.25

The shear modulus is 40833.3332 Pa

Step-by-step explanation:

Given:

Block of gelatin of 120 mm x 120 mm by 40 mm

F = force = 49 N

Displacement = 10 mm

Questions: Find the shear modulus, Sm = ?, shearing stress, Ss = ?, shearing strain​, SS = ?

The shearing stress is defined as the force applied to the block over the projected area, first, it is necessary to calculate the area:

A = 40*120 = 4800 mm² = 0.0048 m²

The shearing stress:

`Ss=(F)/(A) =(49)/(0.0048) =10208.3333Pa`

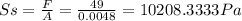
The shearing strain is defined as the tangent of the displacement that the block over its length:

`SS=tan\theta =(Displacement)/(L) =(10)/(40) =0.25`

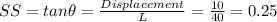
Finally, the shear modulus is the division of the shearing stress over the shearing strain:

`Sm=(10208.3333)/(0.25) =40833.3332Pa`