Question

A psychological study found that men who were distance runners lived, on average, five years longer than those who were not distance runners. The study was conducted using a random sample of 50 men who were distance runners and an independent random sample of 30 men who were not distance runners. The men who were distance runners lived to be 84.2 years old, on average, with a standard deviation of 10.2 years. The men who were not distance runners lived to be 79.2 years old, on average, with a standard deviation of 6.8 years.

Answer 1

Answer:C

Explanation:

Answer 2

1. There is not enough evidence to support the claim that men who were distance runners lived, on average, five years longer than those who were not distance runners.

2. The test statistic is t.

Explanation:

The question is incomplete:

1. Test the claim that men who were distance runners lived, on average, five years longer than those who were not distance runners.
2. Which of the following is the test statistic for the appropriate test to determine if men who are distance runners live significantly longer, on average, than men who are not distance runners?

The test statistic is t, as this is a t-test for the difference of means.

The claim is that men who were distance runners lived, on average, five years longer than those who were not distance runners.

Then, the null and alternative hypothesis are:

H_0: \mu_1-\mu_2=5\\\\H_a:\mu_1-\mu_2> 5

The significance level is 0.05.

The sample 1, of size n1=50 has a mean of 84.2 and a standard deviation of 10.2.

The sample 1, of size n1=30 has a mean of 79.2 and a standard deviation of 6.8.

The difference between sample means is Md=5.

`M_d=M_1-M_2=84.2-79.2=5`

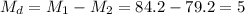
The estimated standard error of the difference between means is computed using the formula:

`s_(M_d)=\sqrt{(\sigma_1^2)/(n_1)+(\sigma_2^2)/(n_2)}=\sqrt{(10.2^2)/(50)+(6.8^2)/(30)}\\\\\\s_(M_d)=√(2.081+1.541)=√(3.622)=1.903`

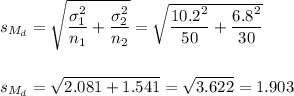
Then, we can calculate the t-statistic as:

`t=(M_d-(\mu_1-\mu_2))/(s_(M_d))=(5-5)/(1.903)=(0)/(1.903)=0`

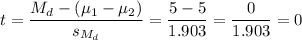
The degrees of freedom for this test are:

`df=n_1+n_2-1=50+30-2=78`

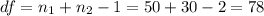
This test is a right-tailed test, with 78 degrees of freedom and t=0, so the P-value for this test is calculated as (using a t-table):

`P-value=P(t>0)=0.5`

As the P-value (0.5) is bigger than the significance level (0.05), the effect is not significant.

The null hypothesis failed to be rejected.

There is not enough evidence to support the claim that men who were distance runners lived, on average, five years longer than those who were not distance runners.