Question

To reduce the drag coefficient and thus to improve the fuel efficiency, the frontal area of a car is to be reduced. Determine the amount of fuel and money saved per year as a result of reducing the frontal area from 20 to 13 ft2 . Assume the car is driven 12,000 mi a year at an average speed of 55 mi/h. Take the density and price of gasoline to be 50 lbm/ft3 and $3.10/gal, respectively; the density of air to be 0.075 lbm/ft3 , the heating value of gasoline to be 20,000 Btu/lbm; and the overall efficiency of the drive train to be 30 percent.

Answer 1

Amount reduction is 29.06 gal/year

Cost of reduction is $90.09 / year

Step-by-step explanation:

Assume The effect of reduction of the frontal area on the drag coefficient is

negligible

Given that:

The drag coefficient (
`C_D`) = 0.4 for a passenger car.

A = frontal area of car = 18 ft²

Velocity (V) = 55 mile per hour = (55 × 1.4667) ft/s = 80.6685 ft/s, density of air (ρ) = 0.075 lbm/ft³

The frontal drag force can be calculated by:

`F_D=C_DA(\rho V^2)/(2) =0.3 *18*(0.075*80.6685^2)/(2) =1317.75\\F_D=1317.75lbm.ft/s^2=(1317,75*(1)/(32.2))lbf=40.9lbf`

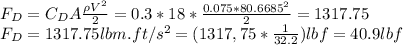
The amount of work done to overcome this drag force is calculated by:

d = 12000 miles per year

`W_(DRAG)=F_D*d=(40.9lbf)*(12000miles/year)*(5280ft)/(1mile)*(1lbf)/(778.169 lbf ft) =3.330* 10^6 Btu/year`

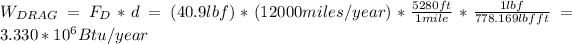
and the required energy input is:

`E_(in)=(W_(DRAG))/(\eta_(car)) =(3.330*10^6Btu/year)/(0.30) =11.1*10^7Btu/year`

Heating value (HV) = 20000 Btu/lbm

`m_(fuel)=E_(in)/HV=1.11*10^7/20000\\`

Amount of fuel =
`\frac{m_(fuel)}{\rho{fuel}} = (1.11*10^7/20000)/(50)=11.1ft^3/year *(7.4804gal)/(1ft^3)=83.03 gal/year`

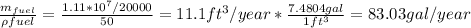
Cost of fuel = Amount of fuel × Price of fuel = 11.1 ft³/year × ($3.1/gal) × (7.4804 gal / 1 ft³) = $257.4 per year.

The percent reduction in the fuel consumption due to reducing frontal area (reduction ratio) is given by:

Reduction ratio =
`\frac{A-A{new}}{A}=(20-13)/(20) =0.35`

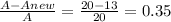
Amount reduction = Reduction ratio × Amount of fuel = 0.35 × 83.03 gal/year =29.06 gal/year

Cost of reduction = Reduction ratio × cost of fuel = 0.35 × $257.4 = $90.09 / year