Question

Radhika ,Supriya and Hema were asked to find the mass of a metal piece independently one by one using the same balance and same weight box. Each student was asked to take three observations. The students reported their result as shown in the following table:

Students Result reported Average
I II III
Radikha 1.325 1.326 1.325 1.325
Supriya 1.310 1.311 1.321 1.314
Hema 1.31 1.30 1.31 1.31
If the actual mass of the metal piece is 1.325g and the permitted uncertainty in the measurement is 0.001g, answer the following questions:
(i) Whose measurement is both accurate and precise? Justify your answer.
(ii) Whose measurement is precise and not accurate Justify your answer?
(iii) Whose measurements are neither accurate nor precise? Justify your answer

Answer 1

i ) Radhika

ii ) Supriya

iii) Hema

Step-by-step explanation:

Solution:-

- Radhika ,Supriya and Hema were asked to find the mass of a metal piece independently one by one using the same balance and same weight box.

- Each student was asked to take three observations. The students reported their result as shown in the following table:

1st 2nd 3rd 4th

Radikha 1.325 1.326 1.325 1.325

Supriya 1.310 1.311 1.321 1.314

Hema 1.31 1.30 1.31 1.31

- The actual mass, u = 1.325 g , permitted uncertainty = 0.001 g

- We will use the data given and determine true important parameters for each student. These are mean value (X,Y or Z) and standard deviation ( Sx,Sy or Sz ). There are n = 4 data points for each student.

Radikha:-

Sample Mean ( X )

`X = (Sum ( X_i ))/(n) = (1.325 + 1.326 + 1.325 + 1.325)/(4) \\\\X = (5.301)/(4)\\\\X = 1.32525`

Standard deviation ( Sx )

`S_x^2 = (Sum ( xi - X)^2)/(n) = ( ( 1.325 - 1.32525)^2 + ( 1.326 - 1.32525)^2 + ( 1.325 - 1.32525)^2 + ( 1.325 - 1.32525)^2)/(4) \\\\\\S_x^2 = 0.00000075\\\\S_x = 0.00086`

Supriya:-

Sample Mean ( Y )

`Y = (Sum ( y_i ))/(n) = (1.310 + 1.311 + 1.321 + 1.314)/(4) \\\\Y = (5.256)/(4)\\\\Y = 1.314`

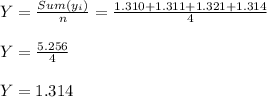
Standard deviation ( Sy )

`S_y^2 = (Sum ( yi - Y)^2)/(n) = ( ( 1.310 - 1.314)^2 + ( 1.311 - 1.314)^2 + ( 1.321 - 1.314)^2 + ( 1.314 - 1.314)^2)/(4) \\\\\\S_y^2 = 0.000074\\\\S_y = 0.00860`

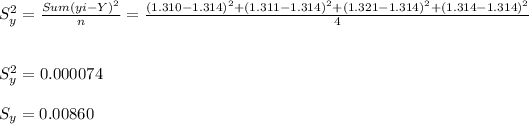
Hema:-

Sample Mean ( Z )

`Z = (Sum ( z_i ))/(n) = (1.31 + 1.30 + 1.31 + 1.31)/(4) \\\\Z = (5.23)/(4)\\\\Z = 1.3075`

Standard deviation ( Sz )

`S_z^2 = (Sum ( zi - Z)^2)/(n) = ( ( 1.31 - 1.3075)^2 + ( 1.30 - 1.3075)^2 + ( 1.31 - 1.3075)^2 + ( 1.31 - 1.3075)^2)/(4) \\\\\\S_z^2 = 0.000075\\\\S_z = 0.05068`

- Now, we will compare the sample mean and sample standard deviation for each student with the actual mass of metal piece and the permitted uncertainty.

Radhika:- X = 1.32525 , Sx = 0.00086 grams.

- The actual mass and sample average mass are close to 4th decimal place making it the most accurate measurement out of all.

- The standard deviation ( Sx ) is the uncertainty in the measurements taken = 0.00086 is well within the permitted allowance of 0.001 grams. Hence, the most precise and accurate measurements were taken by Radhika.

Supriya:- Y = 1.314 , Sy = 0.0086 grams.

- The actual mass and sample average mass are off by:

Error = ( 1.325 - 1.314 )*100 / 1.325

= 0.83 %

- Not as accurate but,

- The standard deviation ( Sy ) is the uncertainty in the measurements taken = 0.0086 is well good around the permitted allowance of 0.001 grams. Hence, her measurement were not that accurate but had good precision.

Hema:- Z = 1.3075 , Sz = 0.05068 grams.

- The actual mass and sample average mass are off by:

Error = ( 1.325 - 1.3075 )*100 / 1.325

= 1.32 %

- Least accurate and,

- The standard deviation ( Sy ) is the uncertainty in the measurements taken = 0.05068 is poor for the permitted allowance of 0.001 grams. Hence, her measurement were neither accurate nor had good precision .

Answer 2

a) Radikha

b) Hema

c) Supriya

Step-by-step explanation:

PRECISION measures the difference or closeness of two or more observed values while ACCURACY measures the difference between the sets of observations to a standard or a given value.

If the measured observation(s) are close to the standard value, then we say that the observations are accurate.

If the values within the ranges of observations are close, then we say the values are precise.

Given the reported value of masses of metal piece as measured by the students:

Result reported Average

Radikha 1.325, 1.326, 1.325, 1.325

Supriya 1.310, 1.311, 1.321, 1.314

Hema 1.31, 1.30, 1.31, 1.31

Actual mass of metal piece = 1.325g

Permitted uncertainty in measurement = 0.001g

a) Based on the observations compared to the standard value, it is seen that Rhadikha measurement is ACCURATE AND PRECISE. It is accurate because each of her observation are all close to the actual mass of the metal piece which is 1.325g, the only value that differs (1.326) vary with just 0.001g which is within the permitted uncertainty in measurement.

Her measurement is also PRECISE because the difference in the values of all observations are also within the permitted uncertainty in measurement which is 0.001g

b) Hema observations is NOT ACCURATE but it is PRECISE. The values are not accurate because the measured observations are not close to the actual mass of the metal based on the permitted uncertainty in measurement.

The values are precise though because the difference in the observations are close and are almost the same.

c) Supriya observations are not accurate and not precise. This is because the measured observations are not close to the actual mass of the metal and the difference in the observations values are not within the permitted uncertainty in measurement.

For example 1.321 - 1.311 = 0.010g which is more than 0.001g.