Question

The diagram shows one way to develop the formula for the area of a circle. Pieces of a circle with radius r are rearranged to create a shape that resembles a parallelogram.

Since the circumference of the circle can be represented by 2πr, and the area of a parallelogram is determined using A = bh, which represents the approximate area of the parallelogram-like figure?

a.A = (2πr)(r)
b.A = (2πr)(2r)
c.A =(2πr)(r)
d.A =(2πr)(r2)

Answer 1

C. A = 1/2(2πr)(r)

Explanation:

The circumference of the circle, 2πr, is the measure completely around the circle. When the pieces of the circle are rearranged, half of this circumference will be on the top of the parallelogram and half will be on the bottom. This means the base will be 1/2(2πr).

The approximate height of the parallelogram is the radius of the circle; this makes the area.

A = 1/2(2πr)(r)

Answer 2

Question:

a. A = (2πr)(r)

b. A = (2πr)(2r)

c.
`A =(1)/(2) (2\pi r)(r)`

d.
`A =(1)/(2) (2\pi r)(2r)`

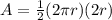
Answer:

The correct option is
`A =(1)/(2) (2\pi r)(r)`

Explanation:

The formula for the area, A of a parallelogram = base, b × height, h

Therefore, since the base dimension is approximately half the perimeter of a circle, we have;

Base, b = 2·π·r × 1/2

Where the height of the parallelogram, as shown in the diagram is approximately the radius of the circle, we have;

Height, h = r

Therefore, the approximate area, A of the parallelogram is given by the following relation;

A = b × h = 2·π·r × 1/2 × r Which is the same as A =
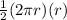`(1)/(2) (2\pi r)(r)`.