Question

Find the shaded region

Answer 1

Answer: ~54.54 units squared, or 25*pi - 24

Explanation:

To find this, we need to find both the area of the triangle and circle, and then subtract the area of the triangle from the area of the circle.

Area of triangle:
`A = (bh)/2`

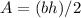
Area of circle:
`A = \pi r^2`

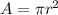
To find the radius, we first need to find the hypotenuse of the triangle, which happens to be the diameter of the circle.

Pythagorean Theorem:
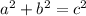`a^2 + b^2 = c^2`, where a and b are sides and c is the hypotenuse.

6^2 + 8^2 = c^2

36 + 64 = 100, c = 10

Knowing that the diameter is 10, we also know that the radius is 5.

Area of the circle with a radius of 5 is 25*pi, or 78.54~

Area of the triangle with side lengths of 6 and 8 is 24.

78.54 - 24 = 54.54 units squared.