We have been given that Carlos needs to take out a loan for 8350 his choice of a five year loan at 5.3% simple interest in a seven year loan at 5.5 simple interest. We are asked to find the difference in the amount of interest charge between two loans.
We will use simple interest formula to solve our given problem.
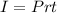 , where,
, where,
I = Amount of interest,
P = Principal amount,
r = Annual interest rate in decimal form,
t = Time in years.

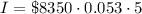
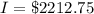
Similarly we will find amount of interest for 7 years at 5.5%.

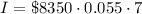

Let us find the difference between both amounts.
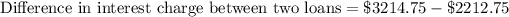

Therefore, the difference in the amount of interest charge between two loans would be $1002.