Question

The city of Oakland is experiencing a movement of its population to the suburbs. At present 85% of the total population lives in the city and 15% of the population lives in the suburbs. Each year 7% of the city people move to the suburbs, while only 1% of the suburb people move back to the city. (a) Write a transition matrix for this information. (b) Write a probability vector for this information. (c) What percent of the population can be expected to be in each category after one year?

Answer 1

A.
`\left[\begin{array}{cc}0.93&0.07&\\0.01&0.99&\end{array}\right]`

B. (0.85 0.15)

C. 79.2% population in the city while 20.8% population in the suburb

Explanation:

(a) The transition matrix for the information is

C S

`\left[\begin{array}{cc}0.93&0.07&\\0.01&0.99&\end{array}\right]`

(b) the probability vector for the information is

`((85)/(100) \ \ (15)/(100) )`

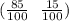
and this gives us

(0.85 0.15)

(c) we simply multiply the above two matrices to find the percent of the population can be expected to be in each category after one year after

`\left[\begin{array}{cc}0.85&0.15\end{array}\right] \left[\begin{array}{cc}0.93&0.07&\\0.01&0.99&\end{array}\right] = \left[\begin{array}{cc}0.792&0.208&\end{array}\right]`

in the city there are 79.2% while in the suburb, there are 20.8%