Question

-Spec, Inc., is attempting to determine whether an existing machine is capable of milling an engine part that has a key specification of 5 ± 0.003 inches. After a trial run on this machine, C-Spec has determined that the machine has a sample mean of 5.002 inches with a standard deviation of 0.002 inch.a. Calculate the Cpk for this machine. (Round your answer to 3 decimal places.) Cpk b. Should C-Spec use this machine to produce this part? Why? (Click to select)YesNo, the machine is (Click to select)capablenot capable of producing the part at the desired quality level.

Answer 1

a)

0.167

b)

No

Step-by-step explanation:

Given that:

The specification limits are 5 ± 0.003 inches, The sample mean (μ) = 5.002 inches, the standard deviation (σ) = 0.002 inch

a)

The interval of the specification is (5 - 0.003, 5 + 0.003 )

The Upper specification limit (USL) = 5 + 0.003 = 5.003 inches

The lower specification limit (LSL) = 5 - 0.003 = 4.997 inches

The formula for Cpk is given by the equation:

`Cpk=min((USL-\mu)/(3\sigma), (\mu-LSL)/(3\sigma))\\ Substituting:\\Cpk=min((5.003-5.002)/(3*0.002),(5.002-4.997)/(3*0.002) )=min(0.167,0.833)=0.167`

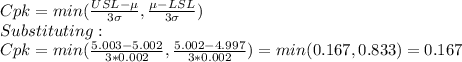
The Cpk is 0.167

b)

No, since the Cpk is less than 1, it means that variation is too wide compared to the specification therefore the machine is not capable of producing the part at the desired quality level.